FAQ on fractal antennas, Part 1

Antennas based on fractal mathematics offer some significant advantages but also some controversy.

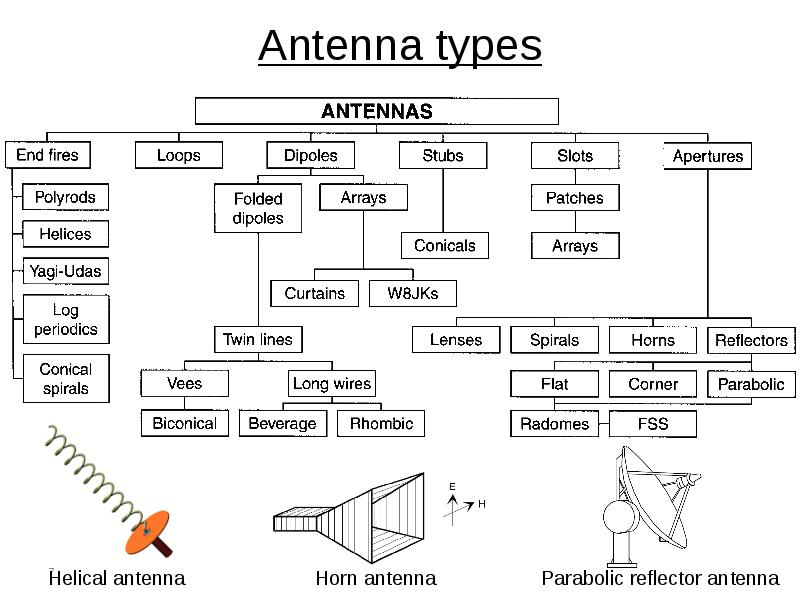
Although all antenna designs are derived from either the basic dipole or monopole/ground-plane configurations, there are almost countless antenna variations in use (Figure 1). Each one offers a different combination of key attributes such as center frequency, bandwidth, bandwidth, side lobes, front-to-back ratio, efficiency, size, cost, ruggedness, and more.

Figure 1. The distinct types and variations on antennas demonstrate that no single configuration is best suited for all applications, but instead, each is tailored to the priorities and constraints of the application (Image: Fayllar.org).

But there’s one antenna implementation that is less well known and often overlooked yet has been designed for applications such as space-constrained smartphones: the fractal antenna. This FAQ will explore the principles behind this antenna and some of the issues associated with it.

Q: What are “fractals,” anyway?
A: Fractals are a class of geometric forms that evolve and replicate internally over multiple iterations. One semi-formal definition is that they are various extremely irregular curves or shapes for which any suitably chosen part is similar in shape to a given larger or smaller part when magnified or reduced to the same size. In colloquial terms, fractals are self-similar whether you view them from close up or very far away; for example, just as a stone at the base of a foothill resembles in miniature the mountain from which it originally tumbled down. They can be based on basic geometrical forms or more complex shapes (Figure 2).

Figure 2. Fractals come in uncountable variations from basic geometrical shapes (top) to those based on repeated curved sets (middle) and even those derived from equations (bottom) (Image sources: New Scientist, Yale University, Wikipedia).

Q: How are fractals generated?
A: They can be generated “by hand,” and there are many examples of fractal images in Hindu temples and Islamic mosques. Now they are often created by applying iterative methods to solving non-linear or polynomial equations.

Q: When were fractals “discovered?”
A: It depends on what you mean by “discovered.” Fractals have been studied by mathematicians informally since the late 1800s, but they did not have a widely presented formal analysis until 1982 when French American mathematician Benoit Mandelbrot published a breakthrough book “The Fractal Geometry of Nature,” (Figure 3) based on his shorter earlier work in French “Les Objets Fractals: Forme, Hasard et Dimension,” as part of his investigation into what he termed the “roughness and self-similarity” in nature.

Q: What did he investigate?
A: He identified that there are some geometric shapes which he called “fractals,” that are equally “rough” at all scales. No matter how close you look, they never get simpler, much as the section of a rocky coastline you can see at your feet looks just as jagged as the stretch you can see from space. In effect, there are smaller and smaller copies of a pattern successively nested inside each other, so that the same intricate shapes appear no matter how much you zoom in to the whole.

Q: Are fractals only conceptual, mathematical constructs?
A: No, they also appear in nature with fern leaves and Romanesque broccoli as just two examples. Of course, many are now generated using computer algorithms and as computers have become more powerful, the intricacy and complexity of the fractals they can generate has increased significantly.

Figure 3. Benoit Mandelbrot’s book not only presented and analyzed fractals but it also brought them to a wider public with its connections between equations, nature, hand-drawn fractals, and computer-constructed images (Image source: Amazon).

Q: Why call them fractals?
A: The term “fractal” was coined by Mandelbrot in 1975. It comes from the Latin fractus, meaning an irregular surface like that of a broken stone or broken glass.

Q: Are fractals discussed extensively in the literature?
A: Since the publication of Mandelbrot’s book, there have been thousands of articles and videos on fractals ranging from artistic to tutorial to mathematically intense works

Q: Is there just one type of fractal?
A: No, they can be divided into various subgroups depending on how they are generated and their “seed” image. One group is named the Mandelbrot Set, but there are many others.

Q: What are the main classes of fractals?
A: Fractals can be classified into two categories: deterministic and random.

Deterministic fractals are generated by iterations using increasingly scaled-down rotated copies of themselves. Such fractals can be generated using computer graphics that are repeatedly mapped out by a recursive algorithm. Random fractals also contain elements of randomness that allow the simulation of natural phenomena. While they relate to nature and are visually fascinating, they are not used for antennas.

Q: What are some examples of deterministic fractals?
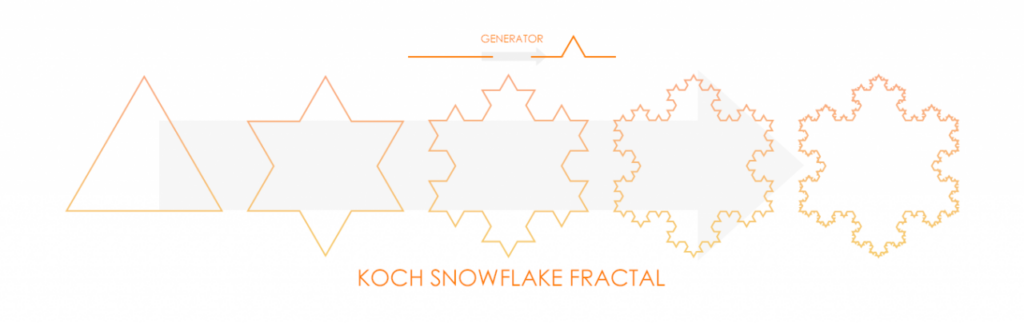
A: Two basic examples are the Koch snowflake (or Koch fractal loop), Figure 4, and the Minkowski Island fractal, Figure 5. The geometry-generating process begins with a basic geometry known as the initiator (the triangle and the square, respectively). For the Koch snowflake, the middle third of each triangle is replaced by a generator, and for the Minkowski Island, each of the four sides of the square is replaced by a generator.